Levelling is the process of finding the height of a new point or points by comparison with that of an existing point which has been selected as a datum or whose height is already known with respect to some other datum. If the points are close to each other and only their relative heights are needed, the simplest procedure is as described in this chapter. If however absolute heights are required, e.g. for comparison with other points elsewhere in the country, then the scheme of levelling should be tied into one or more nearby bench marks1 of known height. (The heights of bench marks around Britain are published by the Ordnance Survey, but cannot now be fully relied upon as many bench marks are no longer checked regularly see Chapter 8.) Alternatively, GPS can be used to find the absolute heights of some of the points, but this is also more complex than might be supposed, as explained in Chapters 7 and 8. The simple process of comparison is generally done with a level and a staff. A level is a telescope mounted on a tripod, fitted with cross hairs and a sensitive bubble; means are provided for setting the line of collimation (the sighting line) to be exactly horizontal. A staff is simply a long ruler and is usually graduated in centimetres.
6.1 Theory
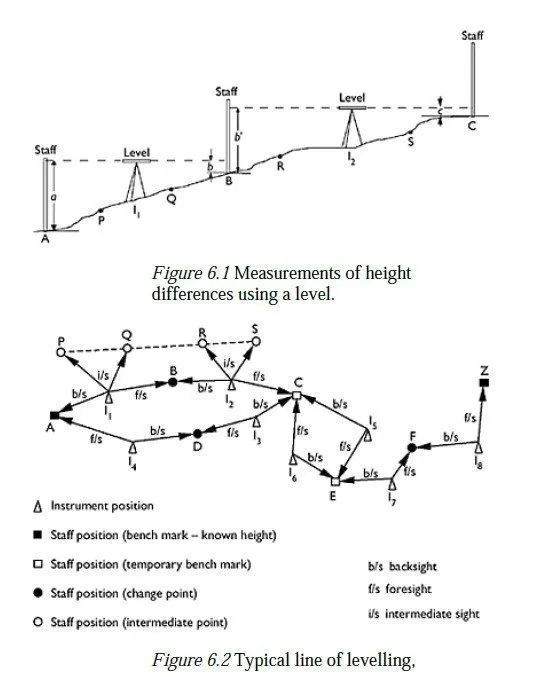
To find the difference in level between two points A and B (see Figures 6.1 and 6.2), the observer sets up the instrument at an arbitrary third point I1. An assistant holds the staff vertical with its foot resting on A. The observer rotates the telescope about its vertical axis until the staff appears in the 1 Typically a horizontal v-shaped slot cut into a vertical wall. They are used by inserting a bracket into the slot and standing a staff on the bracket. The bracket is properly called a bench; hence the term bench mark.centre of the field of view, sets the line of collimation to be horizontal and then reads the scale of the staff against the horizontal cross hair (distance a in Figure 6.1). The staff is then moved to B and the observer again directs the telescope on to it, obtaining the reading b. The difference in level between A and B is then a−b, since, if the instrument is correctly adjusted, both lines of collimation are horizontal. The height of the instrument at I1 does not affect the calculation.
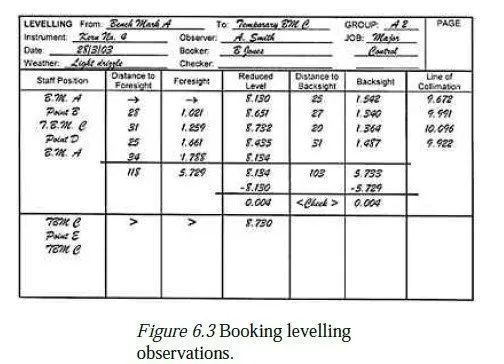
If the height of a third point C, beyond B, is required, the instrument is moved to I2, between B and C. The difference in level between B and C is then found in the same way. By repeating this process, the difference in level between points at any distance apart can be found. In levelling from a mark at A whose level is known (e.g. a bench mark), the observation I1A is called a backsight. This establishes the level of the instrument (or line of collimation) at I1. The observation I1B is called a foresight. Similarly I2B is a backsight, I2C is a foresight, and so on. A line of levelling is usually started at one bench mark of known height and, if possible, finished at a different one. A long line should be broken into a series of bays, of between one and five instrument positions. Each bay should be closed by being levelled out and then back to its starting point, as a check against error. Each bay should run between two well-defined markers which can support the staff and will not change in height; either a permanent bench mark or a temporary one, such as a stout peg. It is helpful if the top of the bench mark is convex or if the peg is driven in at a slight angle, so that there is a uniquely defined highest point on it, which is taken as its height. Figure 6.2 shows a line of levelling in plan view. The first bay runs from a bench mark at A out to C (via B) and then back to A (via D); points B and D are called change points, and point C is a temporary bench mark. A second, smaller bay then runs from C to E and then back to C. Finally, an open bay is run from E to Z; if this gives a height for the closing bench mark which is in good agreement with its published height, then there is no particular need to close that bay back to its starting point, and the calculated heights of all points in the line can be accepted. If the agreement is not good, then the bay should be closed; if it closes well, then it raises the possibility that the first or last bench mark might have subsided since its height was last checked. If the heights of further points are required, the staff is held (say) at P and Q and at R and S, and readings are taken from I1 and I2, respectively. If these intermediate points lie in a straight line and the horizontal distances between them are measured, a vertical section of the ground (a level section) can be plotted. Observations to intermediate points are called intermediate sights; they can be made more quickly than sightings to change points, because several sightings can be made from each instrument position; but they are also more prone to error, because they do not form part of a closed bay.
Note the following:
1 When the staff is moved, the instrument must remain stationary, and when the staff
remains stationary, the instrument must move, to guard against a reading error going
undetected. (If the instrument was not moved from I5 to I6 in the second bay, then a
misreading to the staff at E would cancel out to give a bay which appeared to close
well, yet gave the wrong height for E.) The only time when both move is at the start of
a new bay or at the end of the job.
2 The instrument positions, I1, I2, etc., need not be on the lines AB and BC, etc.
3 It is important that there is something definite to stand the staff on at the temporary
bench marks (positions C and E in Figure 6.2), because both these stations are left and
then revisited later in the job. By contrast, change-point stations B, D and F are only
visited once, so do not necessarily need to be found again later.
4 There is no independent check on the heights recorded for stations P, Q, R and S. Any
point whose height is critical should form part of a levelling line, rather than being
taken as an intermediate sight.
5 A team of experienced levellers might level from bench mark A to bench mark Z
(Figure 6.2) with a single open bay, involving five or more instrument positions. If all
goes well, this is very efficient but if the bay does not close, the whole job must be
repeated. By contrast, a team of novice surveyors would be well advised to make their
first bay as small as possible, such as the one between C and E.
6.2 The instrument
which can be locked by a locking ring. The telescope and a sensitive bubble are pivoted on a horizontal axis and can be slightly elevated or depressed by means of a micrometer screw. There is usually a further cup bubble on the body of the instrument. On setting up, the cup bubble is used to level the instrument approximately by means of the ball joint, but no attempt is made to get the vertical axis truly vertical. Before each individual reading, but after the telescope has been pointed at the staff, the telescope is levelled accurately by means of the sensitive bubble and the micrometer screw. Slopes can also be set out, if the instrument has a graduated ring mounted on the drum of the micrometer screw. In a self-setting or automatic level, a stabiliser automatically levels the line of collimation for every sighting. The stabiliser consists of one prism fixed internally to the telescope casing and two prisms which are suspended freely as a pendulum within the telescope. When setting up, a cup bubble on the tribrach is centred to ensure that the telescope axis is not more than ±20² from the horizontal. The pendulum is then automatically released from its clamps and the staff can be read at once. The makers claim that the pendulum has a repetition accuracy of less than 1 second of arc. This type of instrument saves much time when running a line of levels. It is, however, more subject to interference in windy conditions. At normal ranges (up to about 50 m), both types of level permit readings to be estimated quite easily to within 5 mm. For work requiring greater accuracy, a level fitted with a parallel plate micrometer is used; readings may then be taken to the nearest millimetre. For higher accuracy still, precision levelling can be used (see Section 6.6).
6.3 Technique
Many modern levels (especially precision levels) read the staff digitally; some of the guidance below is specifically for conventional levels, but most is relevant to both types. Set up, focus and eliminate parallax as with a theodolite (see Section 4.3). Level the instrument if necessary, as described above. Never rest your hands on the tripod while observing. We have assumed that the line of collimation is horizontal when the instrument is levelled. This is only true if the instrument is in exact adjustment. If the permanent adjustments are not perfect, the line of collimation will point up (or down) slightly when the instrument is levelled, and all staff readings contain a collimation error. Since this error is proportional to the distance of the staff from the instrument, it will cause equal errors in sights of equal length. Consequently, since the carrying forward of the height depends on the difference between backsights and foresights, the errors will cancel out if, at each position of the instrument, the foresight and backsight distances are equal; i.e. I1A should equal I1B in Figure 6.1. In practice, the errors will be kept small if the difference in distances is less than about 5 m.
The line of collimation can also deviate from the horizontal as a result of the tendency of light paths to bend in a vertical plane (see Section 11.2). The effect of this varies with the square of the length of the line of sight and does not remain constant. With ordinary instruments, therefore, do not take sights longer than 50 m. Particularly when observing on a slope, it is very easy accidentally to use one of the stadia hairs (see Figure 4.1) instead of the main horizontal hair. On a typical sight length of 30 m, this will introduce an error of approximately 15 cm, which should be apparent on checking. It is, of course, preferable not to make the mistake in the first place. On some instruments, the staff is seen upside down in the telescope; do not correct this by holding the staff itself upside down !Before starting work, study the scale carefully, both with the naked eye and through the telescope. Note the difficulty of distinguishing sixes from nines. The staff-holder faces the staff towards the instrument and stands behind it with one hand on each side so as not to hide the scale. It is very difficult to hold the staff vertical. The observer can see if it is leaning sideways by means of the vertical hair in the telescope and can signal to the staff-holder accordingly, but cannot see if it is leaning forwards or backwards. The staff-holder should therefore swing the top of the staff slowly towards and away from the instrument, passing through the vertical position. The observer records the smallest staff reading, since this corresponds with the vertical position of the staff. At change points, rest the foot of the staff on something firm and convex. If necessary drive a peg, preferably with a dome-headed nail driven into it, so that the foot of the staff is always in contact with the highest point, when the staff is vertical. If no dome-headed nail is available, it is actually preferable to deliberately drive the peg in at an angle so that one corner is uppermost, rather than attempting to get the top face of the peg level.
6.4 Booking
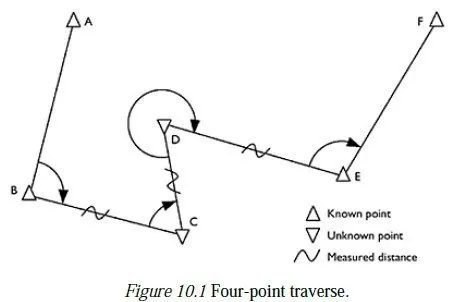
Figure 6.3 shows the method of booking. Successive rows of entries on the form refer to successive staff stations; the foresight from I1 and the backsight from I2 (both to staff position B, in Figure 6.2) are therefore booked on the same line. At each instrument station, the height of the line of collimation is obtained from the backsight, and then the reduced level of the next foresight is calculated by subtracting the relevant staff reading. The booker, who may well also be the observer, books each reading in the field book as it is taken, using a pencil or ball-point pen. Fill in a name for each staff station; the entries in the distance columns need only be approximate and can be judged by pacing. Do not erase; make corrections by drawing a single line through the incorrect figures, leaving them legible and writing the correct figures above them. A fair copy can be made later on another page if necessary, but take care to avoid copying errors, and do not destroy the original papers. Sign and date the work. Immediately after booking a reading, verify it by again looking through the instrument; beware of gross errors of a metre or a tenth of a metre. Then look at the bubble again to verify that the instrument is level.
When the bay is complete, the booker should add up the total of all the backsights (upward movements) and of all the foresights (downward movements), as shown in Figure 6.3. The difference between these two quantities should be the same as the calculated difference in height over the bay (in this case 4 mm). If it is not, it means there is an arithmetic error somewhere on the booking sheet. This check is useful in that it might rescue a bay which appears to have closed badly; conversely, it will also flag a bay which appears to have closed well as a result of two errors cancelling each other. Note, though, that it does not detect errors in the observations themselves. If the bay has closed acceptably and the arithmetic has been checked, the height of all points in the bay can be accepted. If desired, these heights can also be adjusted to their most likely values, given any mis-closure in the bay. In the case shown, point A has closed 4 mm higher than it should; the most likely assumption is that there has been a steady upwards drift around the bay, meaning that the calculated heights for points B, C and D should be adjusted downwards by 1, 2 and 3 mm, respectively. The relevant adjustment is shown for point C in Figure 6.3, as the starting height for the next bay. A further useful check is to add up the total distances for all the foresights and backsights in the bay, as shown in Figure 6.3. In this example, the individual backsights and foresights from each instrument position (25/28, 27/31, 20/25, 31/34) are all individually within tolerance, but there has been a slight systematic bias towards having longer foresights than backsights, as shown by the totals. If the instrument has a collimation error, this accumulating difference will introduce errors into the recorded heights, and might, in this case, explain why the bay has not closed especially well.