Tape Corrections (Surveying) With Example

The following five corrections may be found for the measured lengths of tape:
(i) Corrections for absolute length
(ii) Corrections for pull
(iii) Corrections for temperature
(iv) Corrections for slope and
(v) Corrections for sag.

Corrections for Absolute Length

Corrections for Pull

If pull applied while standardising the length of tape and pull applied in the field are different, this
correction is required.
Let, P0 = Standard pull
P = Pull applied in the field
A = Cross-sectional area of the tape
L = Measured length of line.
E = Youngs modulus of the material of tape, then

Cp = [(P P )L] / AE

The above expression takes care of sign of the correction also.

Correction for Temperature
Let T0 = Temperature at which tape is standardised
Tm = Mean temperature during measurement
α = Coefficient of thermal expansion of the material of the tape and
L = Measured length,
Then the temperature correction Ct is given by,
Ct = Lα (Tm T0)
The above expression takes care of sign of the correction also.

Correction for Slope
If the length measured is L and the difference in the levels of first and the last point is h, then slope correction

Correction for Sag
While measuring on unevenly sloping ground, tapes are suspended at shorter length and horizontal
distances are measured. This technique eliminates errors due to measurement along slopes, but necessitates correction for sag [Fig. 12.23]. Hence, measured length is more than actual length. Thus the correction is ve. The correction, which is difference between the length of catenary and true length is given by
Cs = 1 / 24 (W/P)^2 L

where,
W = the weight of the tape of span length
P = the pull applied and L = measured length
It may be noted that if pull is more than standard pull, the correction for pull is +ve, while correction for sag is always ve. The pull for which these two corrections neutralise each other is called normal tension. Hence normal tension Pn may be found as,
Cp = Cs

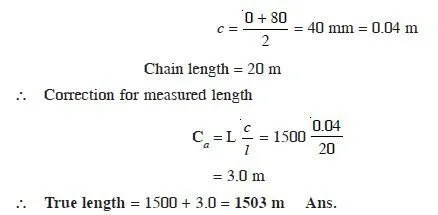
Example 12.2: A distance of 1500 m was measured with a 20 m chain. After the measurement chain was found to be 80 mm longer. If the length of chain was perfectly correct while starting measurement, what is the true length of the line measured?
Solution: Average correction per chain length

Example 12.3: A survey was conducted with a 20 m chain and plan of the field was drawn to a scale of 1 cm = 5 m. The area of the plan was found to be 62.8 cm2. However when the chain was tested at the end of work, it was wound to be 20.10 m. Assuming the length was exactly 20.0 m in the beginning of survey work, determine the true area of the field.
Solution: Initial length of the chain = 20 m

Example 12.4: A 20 m tape was used for measuring a line at an average temperature of 65º F. The
measured distances on the ground and slope of the ground are as given below:
2º 18² for 125 m
3º 30² for 250 m
1º 42² for a distance of 170 m
If the temperature at which tape was standardised is 80º F, find the true length of the line. Take α = 6.2 × 10^(6) /ºF.

Example 12.5: To measure a base line, a steel tape 30 m long, standardised at 15º C with a pull of 80 N was used. Find the correction per tape length, if the temperature at the time of measurement is 25º C and the pull exerted is 150 N.
Take Youngs modulus E = 2 × 10^5 N/mm2 and coefficient of thermal expansion α = 11.2 × 10^6/°C. Cross-sectional area of tape is 8 mm2.
Solution:

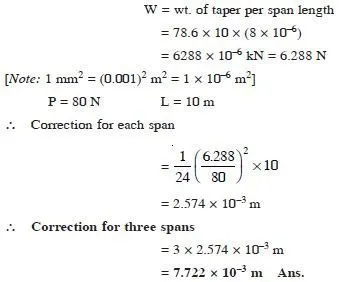
Example 12.6: Calculate sag correction for a 30 m steel tape under a pull of 80 N, if it is suspended in three equal spans. Unit weight of steel is 78.6 kN/m3. Area of cross-section of tape is 8 mm2.
Solution: Length of each span = 10 m