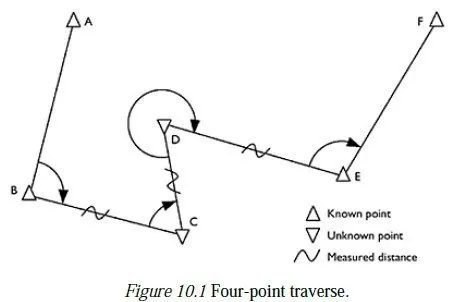
9.6 The realisation of the British National Grid

Section 9.4 has explained how the British National Grid is defined in principle it is important for surveyors in Britain also to understand how it is realised in practice. Between 1936 and 1951, about 480 so-called first-order stations were established around mainland Britain, and their relative positions were found by means of triangulation, plus the measurement of a single base line on the Salisbury Plain. The difficulty of measuring distances accurately and the lack of computing power for adjusting all the observations simultaneously contributed to inaccuracies in the computed positions of those stations which are now apparent with the benefit of modern technology. In the meantime, however, all published mapping in Britain has been based on the grid co-ordinates which were originally published for those stations, collectively known as OSGB36.11 Until the year 2000, the British National Grid was realised (and thus effectively defined) in terms of the physical positions and published co-ordinates of the first-order stations which comprise OSGB36. The true grid positions of some first-order stations, particularly those in northern Scotland, are now known to be up to 20 m different from those quoted in OSGB36. These discrepancies would be sufficient to cause serious confusion over the actual positions of boundaries, etc. compared to their positions as shown on existing maps, and to invalidate many GIS12 databases which use cartographic data from maps based on OSGB36. There is therefore a requirement for the Ordnance Survey to support some kind of transformation between ETRS8913 and OSGB36, so that data collected by GPS equipment can be fully compatible with existing maps and GIS databases.

In response to this requirement, the British Ordnance Survey has developed a transform called OSTN02™ (supplanting the earlier OSTN97™) which converts ETRS89 Cartesian co-ordinates to grid co-ordinates which are very close to OSGB36. The added benefit of this development is that it has allowed the Ordnance Survey to abandon the network of first-order stations as a means of defining the OSGB36 reference frame. Instead, a frame which closely resembles the old OSGB36 is now defined by means of the ETRS89 reference frame, plus the algorithm of OSTN02™. OSTN02™ is freely available in the form of a co-ordinate converter program called GridInQuest, downloadable (April 2003) from the Ordnance Surveys GPS website (https://www.gps.gov.uk/), and is now the standard means for obtaining the grid coordinates of any point whose ETRS89 co-ordinates are known. As such, it complements the OSGM02 National Geoid Model (also included in the converter), which has similarly supplanted the national network of bench marks. The nature of the errors in the original OSGB36 data means that no simple or homogeneous transformation would provide a satisfactory conversion of co-ordinates, so a two-stage process is used. First, the ETRS89 co-ordinates are converted to eastings and northings by applying the transverse Mercator projection and British grid (described above) to the GRS80 ellipsoid. Then, these eastings and northings are converted to OSGB36 eastings and northings using a rubber sheet transformation, which accommodates the many local distortions present in OSGB36 by means of a piecewise bilinear interpolation. The quoted accuracy for this transformation is 0.4 m (for 95 per cent of data), though the relative accuracy between nearby points can be expected to be somewhat better than that.14 The transform is therefore quite accurate enough to map GPS points onto the correct places on an OSGB36 map, but it may cause unacceptable errors in a large engineering project which requires high internal accuracy.

9.7 Coordinate systems for engineering works

As explained above, a simple conversion of GPS data to OSGB36 co-ordinates using OSTN02™ will not produce a fully conformal framework of points. In particular, the scale factor might differ from its theoretical value by up to 20 parts per million and might also depend on the bearing of the line in question. Also, the scale factor changes unpredictably from one region of the country to another, because of the piecewise method originally used to adjust the network of first-order control points. Many engineering works require a higher precision than this, so an alternative method of establishing a fully conformal grid must be used. The possible approaches can be summarised as follows:

1 A simple localised co-ordinate system can be used in areas of up to 5 km2, which do not need to be tied in to any larger system. The method for doing this is described in Section 3.1. Initially, the co-ordinate system would need to be established by conventional means, i.e. total stations and levelling. GPS could be used subsequently, by establishing a one-step transform between the local and (in Europe) the ETRS89 co-ordinates of three control points (see Section 7.6). A scale factor of unity should be used, and the scale factor in the transform should be constrained to be unity, as well.

2 For a larger area, the major control points might be surveyed in by GPS, and the data can be projected directly into UTM co-ordinates on the WGS84 or GRS80 ellipsoid. This can usually be done quite easily in the GPS post-processing software; alternatively, the co-ordinate converter and spreadsheet mentioned above both perform this function for ETRS89 data. Subsequent observations might be made by a mixture of GPS and conventional methods, and the adjustment program LSQ will adjust all the observations to high accuracy, using the scale factor calculations and (t−T) corrections described earlier in this chapter. A geoid model would also be required, to convert the ellipsoidal heights recorded by GPS to the orthometric heights required for the project. This is important, since the local geoid may not be parallel to the WGS84 ellipsoid so differences in ellipsoidal heights (as measured by GPS) may differ significantly from differences in orthometric heights (as measured by a level).

3 If it is necessary to tie in with the British National Grid, a Helmert transform can be set up by quoting the published ETRS89 and OSGB36 co-ordinates of three nearby ETRS89 control points, preferably arranged in a triangle around the area of interest. It is probably wise to constrain the scale factor of the transform to be unity, so that the projections scale factor gives the correct relationship between grid distances and distances on the ground. The accuracy to which transformed points will map onto the OSGB36 system will vary in different areas of the country; an indication will be given by the errors in the three points used to define the transform, which will be reported back by the transformation software. If no geoid model is available, the published orthographic heights of the three points can be quoted as ellipsoidal heights when establishing the transform, and the resulting ellipsoidal heights of further points can then be taken as orthographic heights. Effectively, you are setting up your own special orientation of the Airy ellipsoid, which will provide an even better fit to the local geoid than the standard one.

4 Finally, the Ordnance Survey has published a set of classical transformation parameters to map ETRS89 co-ordinates into OSGB36 (effectively stating the position of the Airy ellipsoid in relation to the ETRS89 ellipsoid). This is a fully conformal transform, which is quoted as having an accuracy of 5 m. The drawbacks of the transform are: (a) that it has a scale factor of 20 parts per million, which would need to be included in all distance computations and (b) unlike OSTN02™ points, these transformed points might have co-ordinates up to 5 m different from those obtained by conventional measurements even to nearby OSGB36 control points. This difference is large enough to make a control point appear to be on the wrong side of a nearby road, when plotted on a map! The parameters of the transform are given in Appendix A.

Overall, it is probably advisable to use option 2, wherever possible. No transformation is involved, so there is no danger of a hidden scale factor. Also, there is no danger of coordinates from different sources, or slightly different transforms, being mistakenly used in the same calculation or adjustment; UTM co-ordinates are sufficiently different from National Grid co-ordinates for their origins to be obvious.