Morphological and morphometric features of relief meso- and macroforms influences on seismic intensity increment.
On basis of the analysis of numerous macroseismic observations the consequences of strong earthquakes, which took place on the territory of the former USSR, S.V.Puchkov and D.V.Garagozov offered the empirical formula for the intensity increment calculation (ΔI) depending on relief feature (Puchkov, Garagozov, 1973):
![]()
where Wgr, Wet are the accelerations of vibratory motion on soil and etalon; Wtop, Wfnd are the accelerations on the top of mountain construction and its foundation.
It was determined as a result of the instrumental and theoretical investigations that for the microrelief the increment of seismic intensity increases from the foundation of mountainshaped feature to its top and can reach approximately 1.8 degree. For the locality mesorelilef the tendency of the increase of seismic vibration intensity from foundation to the top remains. The increment of seismic intensity for the relief mesoforms is about 0.3 degree. It was shown that weak hilly relief, with the inclinations less than 10°, does not influence on the seismic vibrations intensity.
The investigations of S.V.Puchkov and D.V.Garagozov (Puchkov, Garagozov, 1973) showed that at vibrations of mountain range, composed by volcanic tuf, the amplitude of seismic vibrations in S-waves increases on the height 15 m in 1.46 times in comparison with the foundation. For the massif, composed by loamy sand and loams on the same height marks the vibrational amplitude increased in 1.8 times for p-waves and in 3.2 times for S-waves.
Slope steepness considerably influences on the increment of seismic intensity. The increase of slope steepness, composed by incoherent gravel-pebble and sabulous-loamy grounds is conductive to the sharp worsening of engineering-geological and seismic conditions of the territory. So, for example, it is determined that slope steepness more than 19°15° (for dry sandy-argillaceous and gravel-pebble differences) produces the intensity increment up to 1 degree and at variation of slope steepness from 10° to 40° the amplitudes of seismic vibrations increase approximately in 2.5 times.
It is known that the increase of slope steepness from 40° to 80° produces the increment of seismic intensity equal to 1.5 degree (Zaalishvili, Gogmachadze, 1989).
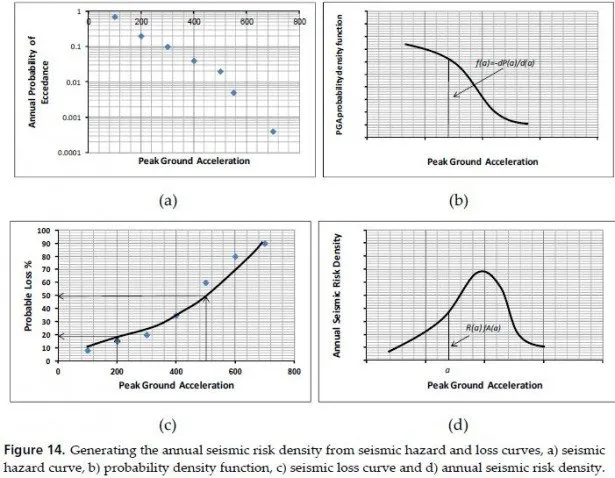
The correlation analysis of the dependence of seismic intensity increment on true altitude, slope steepness and relief roughness showed that the main factors, which change the value of seismic intensity, are the first two indices [Puchkov, Garagozov, 1973]. It conforms well to the investigation results of V.B.Zaalishvili, who introduced the new parameter of the relief coefficient (Zaalishvili, Gogmachadze, 1989) (fig. 14).
Later the data analysis allowed to offer us (I.Gabeeva & V.Zaalishvili) the empirical formula for the possible amplification calculation K and intensity increment ΔI, which are caused by the relief (Zaalishvili, 2006):
K = -0.1 + 0.68lgR

The analysis of the experimental data shows that intensity increment can vary at that independently of the type of rocks, from 0 to 1.5 degree.
Finally, lets try to assess the amplification of vibrational amplitude, which is caused by relief, with the help of the calculation method of FEM (Zaalishvili, 2006).
The algorithm for the calculation of seismic reaction of soil thickness for the twodimensional model was developed for this purpose (fig. 15) (Zaalishvili, 2009). The results of the executed earliear investigations were used for the program testing (Puchkov, Garagozov, 1973). Mountain structure had the form of frustum of a cone with the height 30 m and slope angle of the generatrix 30º. The element maximum size was equal to 5 m, Swave propagation velocity was 300 m/s, the density 1800 kg/m3. The seismic influence was applied to the foundation of soil thickness in the form of instrumental accelerogram, modeling the vertically propagating SH wave.
It was determined that the vibrational amplitude considerably chances with the relief. The given dependence at that is various for the displacements, velocities and accelerations. The largest value of the amplification is observed for displacements and the maximum ratio of vibrational amplitudes, for example, in the point C to the point A, is 2.1 and for the point D 3.2. It well satisfies the results of experimental observations where the ratio in the point C for the S-wave is equal to 2.3 and in the spectral region the maximum values are 1.8 (at T = 0.4 s) and 3.2 (at T = 0.7 s) for P- and S-waves accordingly. Spectral analysis also shows the resonance increase of vibrational amplitudes in the top part of the slope on the frequency 1.6 Hz (i.e. T=0.6 s).

Considerably fewer investigations are dedicated to the influence of the underground relief on the intensity. On the data of B.A.Trifonov (1979) the underground and buried topography of the rocks influences on seismic vibrations intensity, if the surface slope exceeds 0.3. At the vee couch of the rocks, which are covered by sedimentary thickness, the ratio between wave length and the sizes of vee stripping influences on seismic intensity change. Seismic intensity increment in the given case is formed by the wave interference and can be 1.52.0 degree (Bugaev & Kharlov, 1977; Bondarik et.al., 2007).
Thus, at the execution of SMZ works in the mountain regions or under the conditions of billowy relief, it is necessary to pay special attention to the influence of surface or underground relief on the intensity forming. It is necessary to continue the investigations in order to obtain statistically proved ratio for the calculation of intensity increment, caused by
relief.