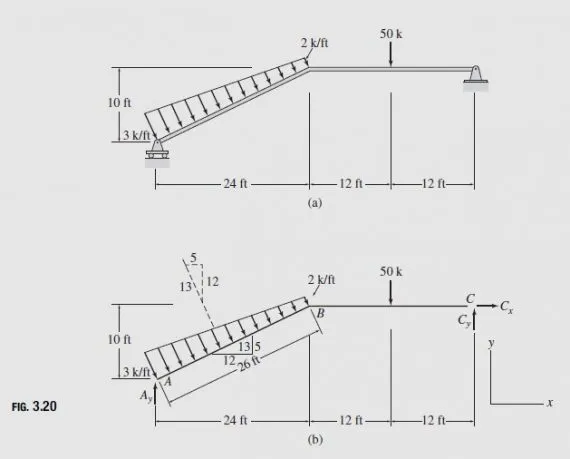
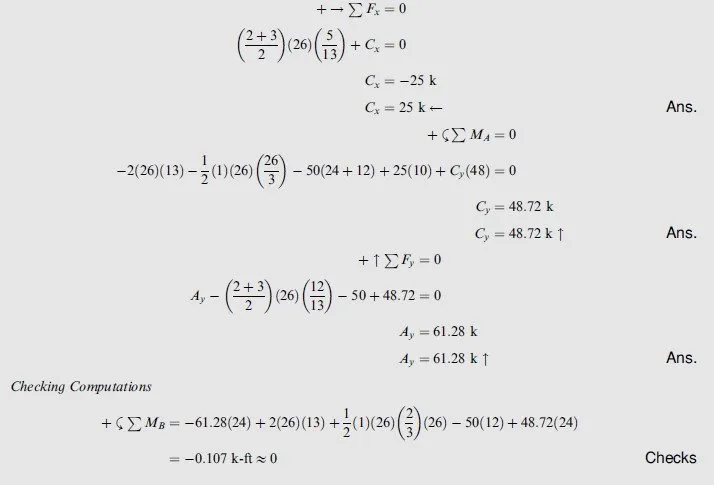
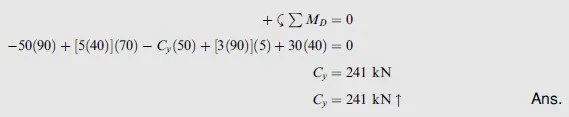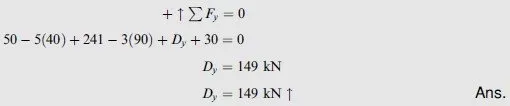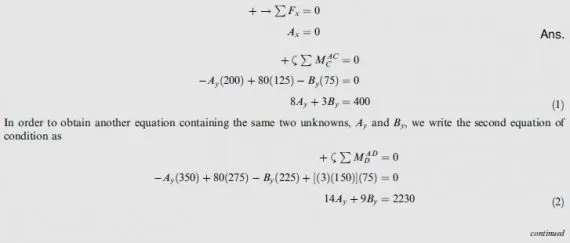Determine the reactions at the supports for the frame shown in Fig. 3.20(a).

Solution
Free-Body Diagram See Fig. 3.20(b).
Static Determinacy The frame is internally stable with r =3. Therefore, it is statically determinate.
Support Reactions

Example 3.7

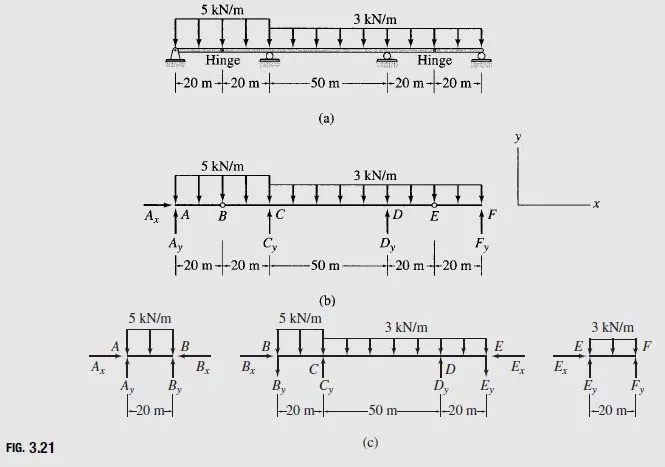
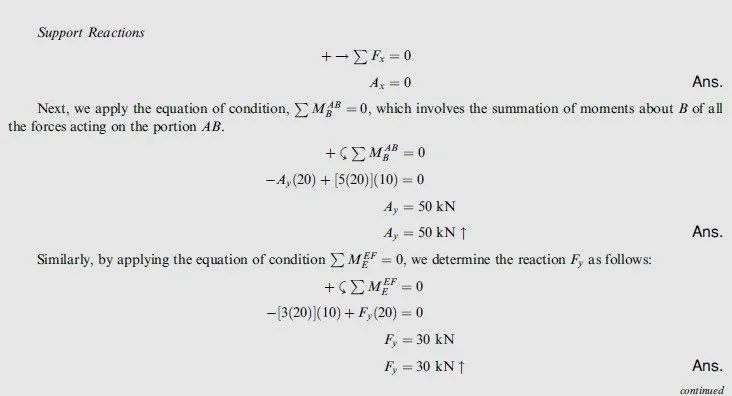
Determine the reactions at the supports for the beam shown in Fig. 3.21(a).

Solution
Free-Body Diagram See Fig. 3.21(b).
Static Determinacy The beam is internally unstable. It is composed of three rigid members, AB;BE, and EF, connected by two internal hinges at B and E. The structure has r =5 and ec = 2; because r =3 + ec, the structure is statically determinate.

The remaining two equilibrium equations can now be applied to determine the remaining two unknowns,
Cy and Dy:

It is important to realize that the moment equilibrium equations involve the moments of all the forces acting on the entire structure, whereas, the moment equations of condition involve only the moments of those forces that act on the portion of the structure on one side of the internal hinge.
Finally, we compute Dy by using the equilibrium equation,

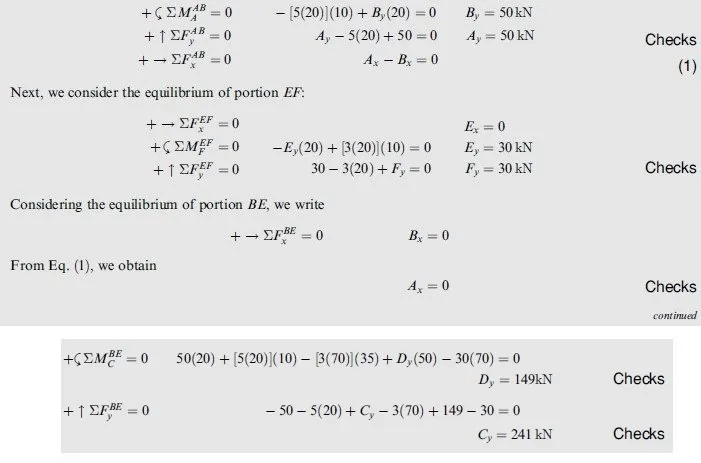
Alternative Method The reactions of the beam can be determined alternatively by applying the three equations of equilibrium to each of the three rigid portions AB, BE, and EF of the beam. The free-body diagrams of these rigid portions are shown in Fig. 3.21 (c). These diagrams show the internal forces being exerted through the internal hinges at B and E in addition to the applied loads and support reactions. Note that the internal forces acting at each end B of portions AB and BE and at each end E of portions BE and EF have the same magnitudes but opposite senses, according to Newtons law of action and reaction.

The total number of unknowns (including the internal forces) is nine. Since there are three equilibrium equations for each of the three rigid portions, the total number of equations available is also nine (r + fi = 3nr = 9). Therefore, all nine unknowns (reactions plus internal forces) can be determined from the equilibrium equations, and the beam is statically determinate. Applying the three equations of equilibrium to portion AB, we obtain the following:

Example 3.8

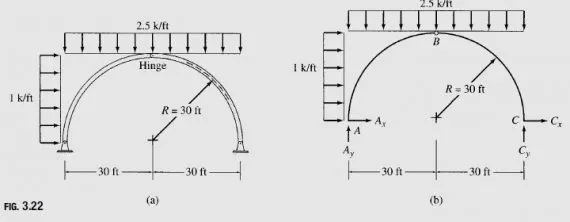
Determine the reactions at the supports for the three-hinged arch shown in Fig. 3.22(a).

Solution
Free-Body Diagram See Fig. 3.22(b).
Static Determinacy The arch is internally unstable; it is composed of two rigid portions, AB and BC, connected by an internal hinge at B. The arch has r =4 and ec =1; since r =3 þ ec, it is statically determinate.
Support Reactions

Example 3.9

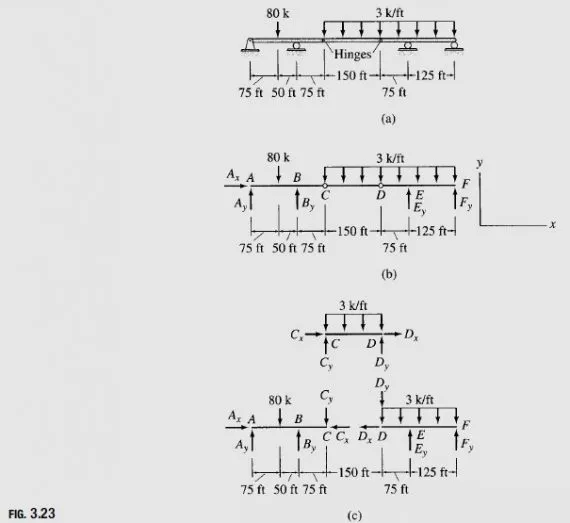
Determine the reactions at the supports for the beam shown in Fig. 3.23(a).,

Solution
Free-Body Diagram The free-body diagram of the entire structure is shown in Fig. 3.23(b).
Static Determinacy The beam is internally unstable, with r ¼ 5 and ec ¼ 2. Since r ¼ 3 þ ec, the structure is  statically determinate.
Support Reactions Using the free-body diagram of the entire beam shown in Fig. 3.23(b), we determine the reactions as follows:

Alternative Method The reactions of the beam also can be evaluated by applying the three equations of equilibrium to each of the three rigid portions, AC;CD, and DF, of the beam. The free-body diagrams of these rigid portions are shown in Fig. 3.23(c). These diagrams show, in addition to the applied loads and support reactions, the internal forces being exerted through the internal hinges at C and D. Applying the three equations of equilibrium to the portion CD, we obtain the following: