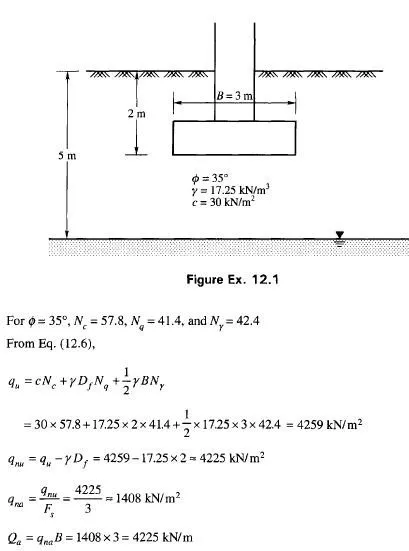
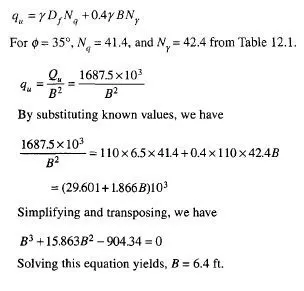The bearing capacity Eq. (12.6) developed by Terzaghi is for a strip footing under general shear failure. Eq. (12.6) has been modified for other types of foundations such as square, circular and rectangular by introducing shape factors. Meyerhof (1963) presented a general bearing capacity equation which takes into account the shape and the inclination of load. The general form of equation suggested by Meyerhof for bearing capacity is
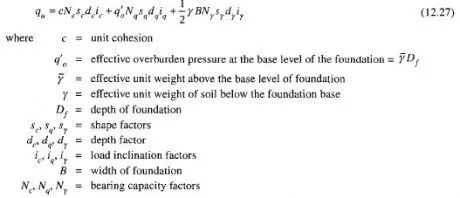
Hansen (1970) extended the work of Meyerhof by including in Eq. (12.27) two additional factors to take care of base tilt and foundations on slopes. Vesic (1973, 1974) used the same form of equation suggested by Hansen. All three investigators use the equations proposed by Prandtl (1921) for computing the values of Nc and Nq wherein the foundation base is assumed as smooth with the angle a = 45° + 0/2 (Fig. 12.6). However, the equations used by them for computing the values of Ny are different. The equations for Nc, Nq and Ny are
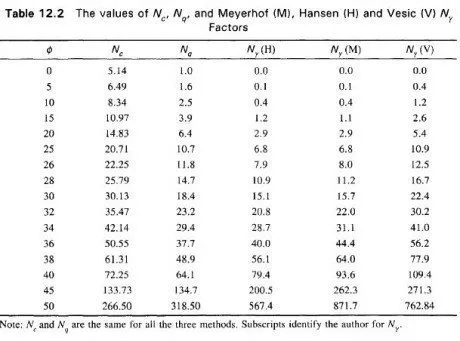

Table 12.2 gives the values of the bearing capacity factors. Equations for shape, depth and inclination factors are given in Table 12.3. The tilt of the base and the foundations on slopes are not considered here.
In Table 12.3 The following terms are defined with regard to the inclination factors
Qh = horizontal component of the inclined load
Qu = vertical component of the inclined load
ca = unit adhesion on the base of the footing
Af = effective contact area of the footing
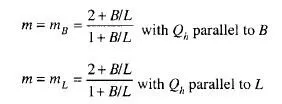
The general bearing capacity Eq. (12.27) has not taken into account the effect of the water table position on the bearing capacity. Hence, Eq. (12.27) has to be modified according to the position of water level in the same way as explained in Section 12.7.
Validity of the Bearing Capacity Equations
There is currently no method of obtaining the ultimate bearing capacity of a foundation other than as an estimate (Bowles, 1996). There has been little experimental verification of any of the methods except by using model footings. Up to a depth of Df~ B the Meyerhof qu is not greatly different from the Terzaghi value (Bowles, 1996). The Terzaghi equations, being the first proposed, have been quite popular with designers. Both the Meyerhof and Hansen methods are widely used. The Vesic method has not been much used. It is a good practice to use at least two methods and compare the computed values of qu. If the two values do not compare well, use a third method.